Electrical engineers use j for the square root of -1 while nearly everyone else uses i. The usual explanation is that EE’s do this because they use i for current. But here’s one advantage to using j that has nothing to do with electrical engineering.
The symbols i, j, and k are used for unit vectors in the directions of the x, y, and z axes respectively. That means that “i” has two different meanings in the real plane, depending on whether you think of it as the vector space spanned by i and j or as complex numbers. But if you use j to represent the imaginary unit, its meaning does not change. Either way it points along the y axis.
Said another way, bold face i and italic i point in different directions But bold face j and italic j both point in the same direction.
Here’s what moving from vectors to complex numbers looks like in math notation:

And here’s what it looks like in electrical engineering notation:
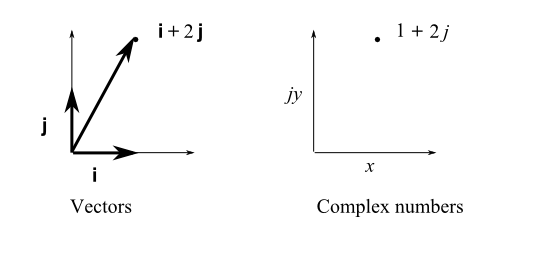
I don’t expect math notation to change, nor would I want it to. I’m happy with i. But using j might make moving between vectors and complex numbers a little easier.
Related: Applied complex analysis

Hey John,
for me it does not make any sense to use j at all. The letter “j” is not within imaginary neither in compley. Choosing “c” for complex numbers would make even more sense to me.
Cheers,
Wolfgang.
Obviously, the solution is to also change the spelling to imajinary. Then everyone’s happy.
@Wolfgang, unfortunately, c is already taken as the speed of light in a vacuum in Physics!
Well i, j and k as components in the 3 dimensional plane came from quaternions, where there is the real component (just a number), plus three others.
vector i and imaginary i are connected, and the same, because the real part was dropped when it was realised that the power of quaternions were in the vector part.
@Tinned_Tuna nobody cares about Physics. Math is there to save the world :-).
Totally agree with Max Bucknell.
Why does Python require j?
Because today’s Tuesday. They use “i” on Mondays.
Kyle: I don’t know. I suppose Guido van Rossum, the language’s creator, was influenced by electrical engineers.
Btw. did you know this:
https://fbcdn-sphotos-g-a.akamaihd.net/hphotos-ak-ash3/529587_464225603626556_217583166_n.png
My E&M prof in grad school, Robert Dicke, argued that j = -i. Just look at how physicists and EEs write a plane wave using complex notation, or how they write Fourier transforms.
In physics the letter ‘j’ is used for the current density, where one also works with complex notation. Thus it is here better to use ‘i’ for the imaginary unit. Because ‘i’ is used for the imaginary unit, ‘i’ is not used for anything else in physics, except for indices etc.
kind regards, Gerhard
Electrical engineering is a heavy user of complex notation, but i was already taken (for current), so the next letter in line got promoted.
Stephen: why did you feel the need to repeat the second sentence of the article as if it were new information?
There’s much more to it. If you read David Hestenes books and papers on the geometric algebra and its application to physics you’ll find i is the unit bivector corresponding to the outer product of x and y in two-dimensional space, just as i, j, and k are the unit bivectors corresponding to the outer products of x and y, y and z, and z and x in three-dimensional space. A bivector is the oriented plane spanned by two vectors which are not in a line. The plain old complex numbers are the even sub-algebra of the two-dimensional geometric algebra, and the plain old quaternions are equivalent to the even sub-algebra of the three-dimensional geometric algebra. Exact correspondences get messed up by different choices of left and right handed rules. And everything can be extended to arbitrary dimension, so we get trivectors (the oriented volume spanned by three vectors that are not in a plane), and so on.
I’ve always just used î to differentiate between the unit vector and other i.
I only used i, j, and k when I was a college freshman and when I taught college freshmen.
In my own work I simply use triples for vectors in R3 and more generally n-tuples for vectors in Rn. I don’t usually use any symbol for basis vectors, but if I need one I use ei for the unit vector in the ith direction.
Were there a lot of Germans in early electrical engineering? I’ve seen Iodine (I) represented as J in German chemistry papers (The German prof I had at the time was less then impressed at his countrymen not obeying IUPAC conventions)
Anyway, apprently some parts of Germany use J for I, could that be involved here?
Why do not use the Greek symbol iota. It has been used in many books for imaginary unit. I do use in my paper when I have to use i to denote some other variable.
I would like to point out another point. I came from a pure math background and entered the EE field. I found it quite annoying to make the transition from i to j. But then one day I had an epiphany that totally changed my perspective on the matter. The fact of the matter is, there is no contradiction between i or j from a pure mathematical perspective when viewed as imaginary units (with no regard to unit vectors as you so cleverly pointed out).
It all has to do with quaternions. In a quaternion number system, we have numbers of the form a + bi + cj + dk, where a,b,c,d are all real and i,j,k are all imaginary units, but the imaginary units do not equal one another. i^2 = j^2 = k^2 = -1. And ijk = -1. But i =/ j =/ k.
Any quaternion whose values c=d=0 reduces to a+bi, and you have a standard complex number. But heres the kicker… any quaternon whose values b=d=0 reduces to a+cj, which is also a pure complex number and behaves exactly as a+bi would and can be analyzed with all the same tools.
So… from a pure mathematical perspective I have come to realize that a+bi and a+cj are both pure complex numbers with all the bells and whistles, but no one ever has to rationalize how or why i=j, because it doesnt even have to be true.
i dont think i is used insted of j just to avoid confusion as i is used for representation of current,what do u say if u get to know that in EEE j is used for AC current density and J is used for DC current density
OCR (Oxford Cambridge and RSA) Uses j for their Further maths A level Courses
Already beaten, but yeah as far as I can tell, Hamilton was the first to use ‘j’ and ‘k’. He needed two additional features to represent orthogonal elements which shared the properties of i. Presumably, he simply due to the ordinal nature of the English language. Here’s Hamilton’s letter (1843) [1]
And of course there’s the famous bridge graffiti: i^2 = j^2 = k^2 = ijk = −1.
IIRC Gauss’ semi-developed version just used the convention of abcd. Here’s a letter from Euler to Goldbach also using abcd [2]
Tangentially, “Geometric Algebra” / “Clifford Algebra” is a way more elegant way to represent EMF, as it reduces Maxwell’s traditional set of 4 to 1. [3] More importantly, however, it eliminates the lack of symmetry as well as the arbitrary chiral nature of fields. Here’s a very approachable blog post[4] .
Anyways, if anyone can beat Hamilton’s 1843, I’d love to hear about it.
-Andrew
[1] http://www.maths.tcd.ie/pub/HistMath/People/Hamilton/QLetter/QLetter.pdf
[2] http://eulerarchive.maa.org//correspondence/letters/OO0841.pdf.
[3] Trivia – Maxwell’s original formulation of the 2 Gauss laws, as well as the Ampere and Faraday laws, were a set of 24 equalities. I’d love to know historically when it was reduced to 4 (which can trivially be reduced to 2 via co-variance — see: simple.wikipedia.org/wiki/Maxwell’s_equations#Covariant_Formulation).
[4] https://slehar.wordpress.com/2014/03/18/clifford-algebra-a-visual-introduction/