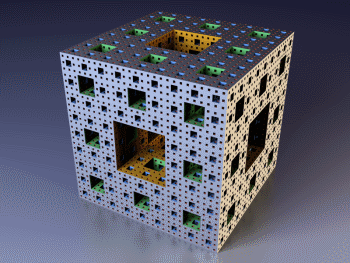
You can make a fractal by starting with a cube and recursively removing chunks of it. The result is called a Menger sponge. Here’s a picture after four levels of recursion.
What would it look like if you sliced this open? If you cut parallel to one of the faces it looks just like the original object. But if you cut at an odd angle, you’d be surprised. Here’s a New York Times article about the result.
Karl Menger came up with his sponge in 1926, but only recently did someone think to slice one open as in the article.

Back in the late 80’s I was doing CFD on unstructured tetrahedral grids.
One task was to write a program (graPhigs & FORTRAN) to view the solution sets, including cut planes through the volume data. I just assumed that the results plotted would be made of triangles.
The first cross section plot of a SR71 at M=3 showed this beautiful pattern of triangles…and all these quadrilateral holes. Very cool. After the first WTF? it took about 30 seconds with paper and pencil to figure out what had happened.
Even after I fixed the error I kept a couple of the original plots, because they were neat, and as a amusing and humbling reminder about assumptions.