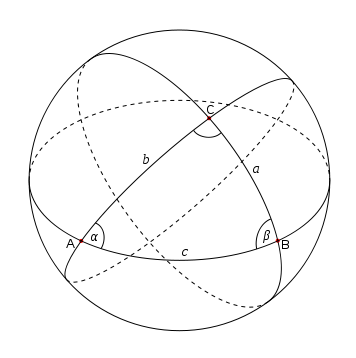
Last spring I wrote a post on spherical trigonometry, the study of triangles drawn on a sphere (e.g. the surface of the Earth).
Mel Hagen left a comment on that post a few days ago saying
I am revisiting Spherical Trig after 30 years by going back over some of my books that I have collected over the years. …
I asked Mel via email why he was revisiting the subject. He wrote an interesting reply that I am including below with his permission.
Mr. Cook,
Well, let’s see, how did I come to revisit the world of Spherical Trigonometry?
In the early 1970’s I lived within a mile of the coast of the Gulf of Mexico in southern Alabama. I took up daytime sailing with some of my friends from work (they sailed — I just went along for the ride).
Eventually I brought up the concern of being out of sight of land and how do we know where we are. In addition to LORAN navigation receiver and a Radio Direction Finder receiver they always had at least two navigation sextants on board. They demonstrated very quickly and without much detail how to get a “fix.”
I never thought much about it after that until I was at a yard sale in our town and there was a small book, Celestial Navigation for Yachtsmen by Mary Blewitt. I spent the dollar, took it home and spent the next year or so reading it over and over until I had a idea of what was really going on with simple calculations and the examples using tables and charts.
Having taken most of the mathematics courses available in three different colleges I knew there had to be strong basis for Spherical Trigonometry in Celestial Navigation.
Anyway, her book deals exclusively with finding out where you are but not how to point yourself with the true heading for where you want to go. So I dug out several of my old textbooks including the Schaum’s Outline Series by Frank Ayres, Jr., and found just what I was looking for.
Now, let me digress for a moment. In the art of Celestial Navigation you really need three important items: the sextant, a reasonably accurate watch and either a Nautical Almanac or an Air Navigation Almanac. Nowadays you can buy a digital watch for less than $30.00 that keeps time better than anything they had during World War II. With those 3 items and some simple Spherical Trigonometry you can easily determine your location on the earth (Law of Sines and Law of Cosines).
So, time for a case scenario.
You’re out to sea. A storm comes up. The vessel loses all electronic instrumentation (radios, compasses, computers, LORAN, GPS, etc.). (If you think this doesn’t happen in real life, think again!) But, as long as you can see the sun during the day you’re in better shape than you think.
Most important — you must have at least one watch that is set for Greenwich Mean Time!
As it is getting about mid-day you start taking sextant sights of the Sun. when it reaches its peak you write down your watch time. With 15 degrees per hour from midnight on your watch you now have local time. With the almanac you can get the declination of the Sun. You should have a reasonable idea of where you think you are. Combine that with two or three Spherical Trigonometry calculations and you can get your “fix.”
Knowing where you are and where you should be headed and using Napier’s Analogies, you can determine where you should point your vessel and get on you way. With a few more calculations you can actually determine how many hours of daylight you have left — and — when the sun will come up in the morning to give you a good reference check.
So curiosity got the best of me. I started playing with Spherical Trigonometry, logarithm tables, and a very good slide rule all over again. The name of the game is not to just use the formulas and the equations but derive them so that you don’t have to try to memorize them and make a mental translation error.
I think one of the things we are doing today is forgetting how we got where we are using certain fields of mathematics and relying too heavily on technology that can easily fail us. Spherical Trigonometry is just an extension of 1 plus 1 equals 2. It just takes some reading and practice, practice, practice.
By the way, another book (if you can find it) that is really helpful is, Standard Mathematical Tables.
Keep in touch. Let me know how people respond to my background and also the sources of information.
Mel Hagen
mbhagen@yahoo.com

That is really interesting. It gave me some ideas.. These days, smartphones (iPhone, Android phones, etc..) already have all that’s needed; They have accurate clocks, they can store all the charts needed, they have sensors to measure the angle they’re tilted at, they have cameras to see the sun, and they can do millions of calculations per second. Someone should write an app for that.
Yes, they also have GPS, but sometimes you don’t have signal. It’s also an interesting app to show to your friends.
This person may have granted you permission to quote them, but did they intend for you to also post their contact information?