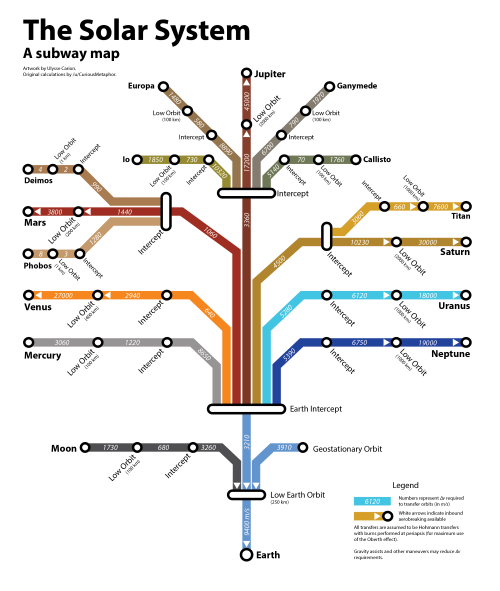
This is a thumbnail version of a large, high-resolution image by Ulysse Carion. Thanks to Aleksey Shipilëv (@shipilev) for pointing it out.
It’s hard to see in the thumbnail, but the map gives the change in velocity needed at each branch point. You can find the full 2239 x 2725 pixel image here or click on the thumbnail above.

Very nice, but shouldn’t it be Supway, rather than Subway?
Awesome! Now make one for Kerbal Space Program.
For KSP, the subway map is here: http://wiki.kerbalspaceprogram.com/wiki/Cheat_sheet#Maps
The 27 km/s edge to the surface of Venus seems out of whack when compared with the other equivalent edges.
Hmmm. I ran across this:
https://mobile.twitter.com/search?src=typd&q=%23drunktufte
over at flowingdata.com and it gave me courage to say that I don’t really think the map above is a useful tool.
The point to the original map was to make the things the rider needed – stations and their order clear – minor bends and exact distances were clutter.
Carion’s map uses a similar format & colors but the most important thing, the delta-V, is TEXT (you had to point it out). The visual display of the most important piece of quantitative information is entirely lacking!
Plucky, Venus has about the same escape velocity as Earth if you ignore the atmosphere, but the atmosphere is extremely thick (92 atm at the surface, and meaningful density up to 250km, compared to 100km for Earth), which wreaks havoc on ascents. Getting down to the surface isn’t nearly as difficult since you can use the atmosphere itself to do the work by aerobraking; the trouble then is just surviving the intense pressure and heat you find when you get there.
That means it’s pretty much pick-a-number, then. You could probably aerobrake to near-rest at the 0.1 atm level and parachute the rest of the way; what’s the delta-V associated with that? The arrows point down, not up. There’s at least one other map on the Web that adds a mere notional 2km/s to get from (not to) the surface of Venus. And what level and/or direction do you use for the gas giants?
This isn’t in any sense a fundamental criticism of the map — it’s fascinating — but a one-line addition to the legend, stating the basis for computation and the exceptions, would make it even better. I’ll have a try at contacting the author.
Plucky, the point is that on bodies with atmospheres, the deltaV listed on the map is for an ascent, because a descent can be arbitrarily close to 0. For bodies without atmospheres, ascent and descent have the same cost, so the number applies to both.
The directions the arrows point indicate that it’s easier to move “with” the arrow than “against” it; the arrows aren’t attached to the numbers.
OK, that’s consistent with the Wikipedia page on the dynamics of LEO (7.8 km/s “pure” delta-V plus 1.6 — not so sure what Wikipedia means by “gravity drag” unless 7.8 is the orbital speed at altitude rather than at surface in a notional vacuum).
Gravity drag is the velocity lost due to the acceleration from gravity towards the body you’re leaving. It’s the reason why a vessel with a TWR of 2 will have an acceleration straight up of 1 g, and a vessel with a TWR of 1 or less won’t get off the ground at all :)
The total loss to gravity drag is approximate because it ends up depending on TWR, ascent profile, aerodynamics, staging, and other variables — in short, the less time you can spend on the ascent phase and the sooner you can get your velocity substantially horizontal, the lower gravity drag will be. Wikipedia’s page on the topic is a decent enough overview.