Yesterday my daughter came by and dropped off a huge blue wine bottle (empty).

She had started removing the label, but as you can see she didn’t get very far yet. It’s an Incanto Chardonnay Pinot Grigio from Trader Joe’s.
I blew across the top of the bottle to hear what sound it makes, and it makes a nice deep rumble.
I tried to identify the pitch using a spectrum analyzer app on my phone, and it says 63 Hz.
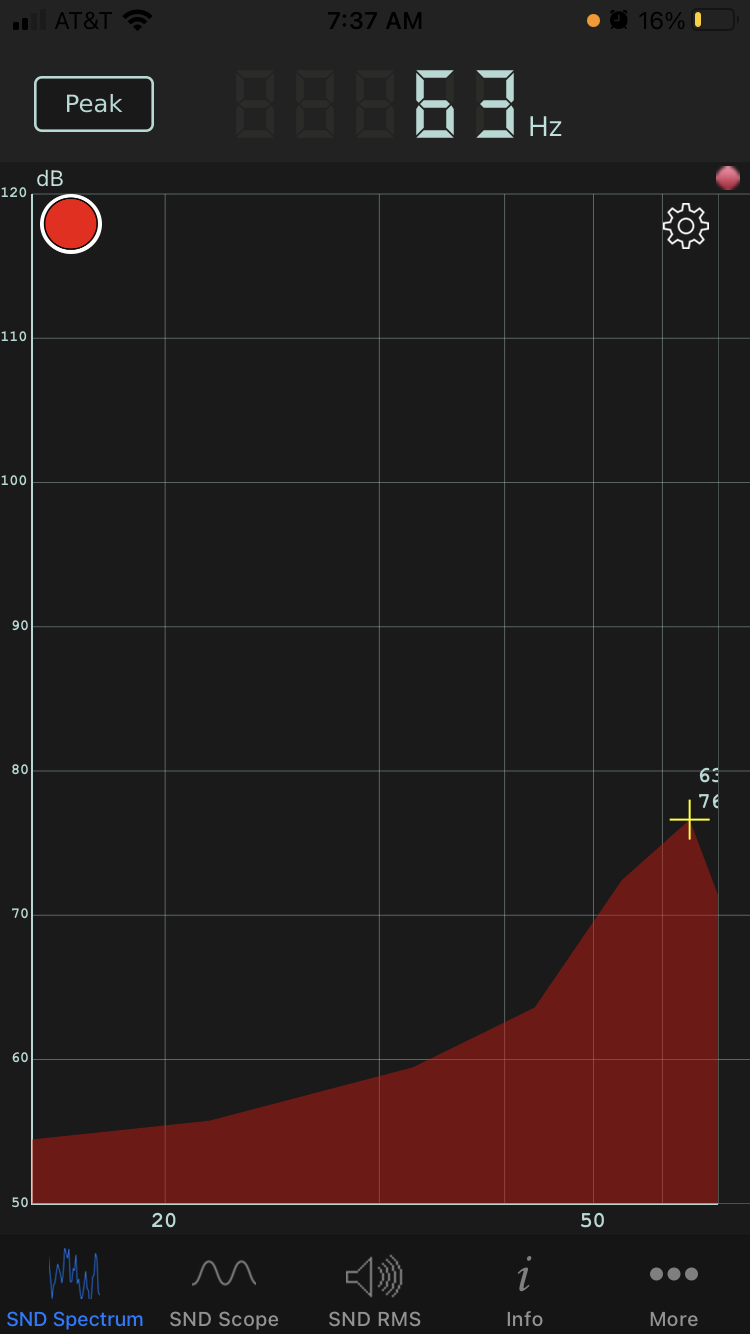
Next I tried to figure out what pitch I should expect theoretically based on physics. Wine bottles are Helmholtz resonators, and there’s a formula for the fundamental frequency of Helmholtz resonators:
The variables in this equation are:
- f, frequency in Hz
- v, velocity of sound
- A, area of the opening
- L, length of the neck
- V, volume
I measured the opening to be 3/4 of an inch across, and the neck to be about 7 inches. The volume is 1.5 liters. The speed of sound at sea level and room temperature is 343 meters per second. After a few unit conversions [1] I got a result of 56.4 Hz, about 10% lower than what the spectrum analyzer measured.
An ideal Helmholtz resonator has a cylindrical neck attached to a spherical body. This bottle is far from spherical. The base is an ellipse with a major axis about twice as long as the minor axis. And from there it tapers off more like a cone than a sphere [2]. And yet the frequency predicted by Helmholtz’ formula comes fairly close to what I measured empirically.
I suspect I got lucky to some extent. I didn’t measure the bottle that accurately; it’s hard to even say when the neck of the bottle stops. But apparently Helmholtz’ formula is robust to changes in shape.
Update: Pitch of a beer bottle
I repeated my experiment with a beer bottle, specifically a Black Venom Imperial Stout.

The opening diameter is about 3/4″, as with the wine bottle above, and the neck is about 3″ long. The volume is 12 fluid ounces. Helmholtz’ formula predicts a pitch of 177 Hz. My spectrum analyzer measured 191 Hz, the G below middle C. So this time theory was about 7% lower than the observed value.
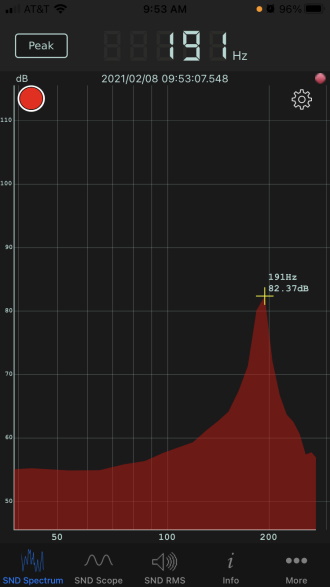
The beer bottle is closer to the shape of a Helmholtz resonator than the wine bottle was. It’s at least radially symmetric, but the body is a cylinder rather than a sphere.
Update 2: Typical wine bottle
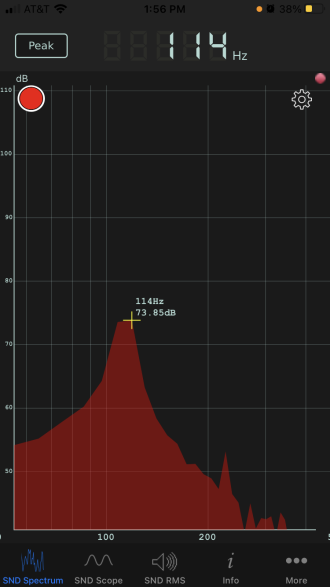
When I tested a typical 750 ml wine bottle, I got a pitch of 114 Hz. With a 3.5 inch neck and a 0.75 in diameter opening, the calculated pitch was 113 Hz. There’s some element of luck that theory and measurement agree so well, especially since the punt at the bottom means its shape is even further from spherical than that of a beer bottle.
More acoustics posts
[1] Thanks to a reader who provided this write-up of the calculation:
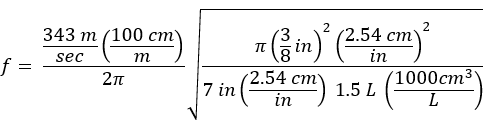
[2] What we usually call a cone is more specifically a right circular cone. But more generally a cone can have any base, not just a circle, and this bottle is approximately an elliptical cone.

Your phone’s spectrum analyzer app seems to be very good.
What’s the app’s name on Google Play store ?
63Hz is lower than you think. It is ~~the pitch of the bottom string of a ‘cello.
You’re right. I think what threw me off is that the deepest tone is not the loudest component, loud in the technical sense of perceived volume. I think the 63 Hz component is the one with the largest sound pressure even though I hear an overtone more prominently.
@E.Sarmas: The app is called “Sonic Tools.” I’m running it on an iPhone. I don’t know whether it’s available on Android.
The Helmholtz resonator is used in acoustic phonetics. Certain sounds, such as rhotics (r-like sounds), are usefully modeled as Helmholtz resonators coupled to the pipes used to model the vocal tract overall. This is a nice example I found with a quick search, in case you’re curious.
https://www.researchgate.net/publication/228756572_Acoustic_strategies_for_production_of_american_english_retroflexr
Is the shape of the bottle related to the shape of the spectrum, beyond just the peak value, in some computable way? In fact, is there a standard way to parameterise spectra like this, perhaps for some kind of ‘generalised Helmholtz resonator’?
I have to build H resonators to solve a 10dB resonance of my home cinema room in the basement. Problem occurs around the beer bottle frequency. Maybe a collection of tuned bottles can solve the issue! I will defenitely try it ;-)