I was skimming through the book Mathematical Reflections [1] recently. He was discussing a set of generalizations [2] of the Star of David theorem from combinatorics.
The theorem is so named because if you draw a Star of David by connecting points in Pascal’s triangle then each side corresponds to the vertices of a triangle.
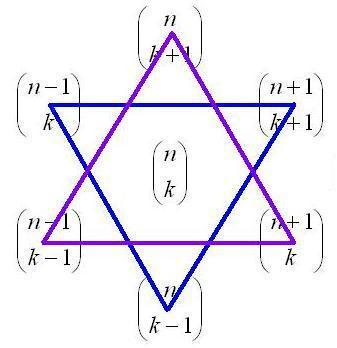
One such theorem was the following.
This theorem also has a geometric interpretation, connecting vertices within Pascal’s triangle.
The authors point out that the binomial coefficient is a separable function of three variables, and that their generalized Star of David theorem is true for any separable function of three variables.
The binomial coefficient C(n, k) is a function of two variables, but you can think of it as a function of three variables: n, k, and n − k. That is
where f(n) = n! and g(k) = 1/k!.
I was surprised to see the term separable function outside of a PDE context. My graduate work was in partial differential equations, and so when I hear separable function my mind goes to separation of variables as a technique for solving PDEs.
Coincidentally, I was looking a separable coordinate systems recently. These are coordinate systems in which the Helmholtz equation can be solved by separable function, i.e. a coordinate system in which the separation of variables technique will work. The Laplacian can take on very different forms in different coordinate systems, and if possible you’d like to choose a coordinate system in which a PDE you care about is separable.
Related posts
- When is a function of two variables separable?
- Justifying separation of variables
- Laplace’s equation in elliptical coordinates
[1] Peter Hilton, Derek Holton, and Jean Pedersen. Mathematical Reflections. Springer, 1996.
[2] Hilton et al refer to a set of theorems as generalizations of the Star of David theorem, but these theorems are not strictly generalizations in the sense that the original theorem is clearly a special case of the generalized theorems. The theorems are related, and I imagine with more effort I could see how to prove the older theorem from the newer ones, but it’s not immediately obvious.
